Fluid Mechanics - Important Points before Exam

Fluid Mechanics

Civil Engineering

Bernoulli’s equation

Assumption

Following assumption are made in deriving the Bernoulli’s equation

• 1) Flow is steady

• 2) Fluid is incompressible

• 3) Fluid is non-viscous (inviscid)

• 4) Equation is applicable along a streamline

• 5) Effect of friction is neglected

• 6) Only pressure and gravity forces are taken into account

• 7) Velocity is uniform over the cross-section

• 8) Surface tension is zero

Total drag = Pressure drag + Viscous drag

Since given fluid is ideal i.e. it has zero viscosity, so viscous or friction drag is zero.

Since sphere is symmetrical in shape and it is subjected to hydrostatic pressure all around the sphere in such a way that net force or pressure drag is zero.

Hence, total drag is always zero when an ideal fluid past through a sphere.

Ideal Fluid

Definition of Ideal Fluid:

An ideal fluid must satisfy the following conditions:

• 1. Frictionless or inviscid fluid.

• 2. In compressible

• 3. No surface tension effect.

Objects in a fluid experience an upward force. Whenever an object is immersed in a fluid, either liquid or gas, it experiences a buoyant force. A buoyant force is a force which pushes upward on an object and is caused by displaced fluid.

The centre of buoyancy is the point where the resultant Buoyant force acts, It is the point of the C.G of the displaced fluid.

Pitot Tube

• It is a device used for calculating the velocity of flow at any point in a pipe or a channel. 
• The pitot tube is used to measure velocity at a point.

• In the question velocity at the stagnation point is given which is zero. So here stagnation pressure will be the correct answer. Because this stagnation pressure head is used to calculate the velocity at a point.

• V = √2gh

• It is based on the principle that if the velocity of flow at a point becomes zero, the pressure there is increased due to the conversion of the kinetic energy into pressure energy. 

Working principle of Pitot tube:

• The liquid flows up the tube and when equilibrium is attained, the liquid reaches a height above the free surface of the water stream
• Since the static pressure, under this situation, is equal to the hydrostatic pressure due to its depth below the free surface, the difference in level between the liquid in the glass tube and the free surface becomes the measure of dynamic pressure 

•  where p0, p and V are the stagnation pressure, static pressure and velocity respectively at point A
• Such a tube is known as a Pitot tube and provides one of the most accurate means of measuring the fluid velocity
• For an open stream of liquid with a free surface, this single tube is sufficient to determine the velocity, but for a fluid flowing through a closed duct, the Pitot tube measures only the stagnation pressure and so the static pressure must be measured separately.

Coefficient of discharge is the ratio of actual discharge to the theoretical discharge.

Coefficient of discharge

• ⇒ Venturimeter – 0.95 to 0.98

• ⇒ Orifice meter – 0.62 to 0.65

• ⇒ Nozzle meter – 0.93 to 0.98

• ∴ Coefficient of discharge for venturi meter lies is higher than the nozzle meter and orifice meter.

Stream Function: 

• It is a scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles (in a counter-clockwise direction) in this direction.

Potential Function: 

• A scalar function of space and time such that its negative derivative with respect to any direction gives the fluid velocity in that direction.

Circulation: 

• It is the line integral of the tangential component of the velocity taken around a closed contour.

Vorticity: 

• The limiting value of circulation divided by the area of closed contour, as the area tends to zero.

∴ The value obtained from dividing the limiting value of circulation by area of closed contour is known as vorticity.

Euler's equation

• The Euler's equation for a steady flow of an ideal fluid along a streamline is a relation between the velocity, pressure and density of a moving fluid. It is based on the Newton's Second Law of Motion. The integration of the equation gives Bernoulli's equation in the form of energy per unit weight of the following fluid.

It is based on the following assumptions:

• The fluid is non - viscous (i,e., the frictional losses are zero)
• The fluid is homogeneous and incompressible (i.e., the mass density of the fluid is constant)
• The flow is continuous, steady and along the streamline
• The velocity of the flow is uniform over the section
• No energy or force (except gravity and pressure forces) is involved in the flow

Uniform Flow, Steady flow, Steady-Uniform flow

• The flow is defined as uniform flow when in the flow field the velocity and other hydrodynamic parameters do not change from point to point at any instant of time.
• Steady flow, a flow in which the velocity at any point in the channel does not change with time.
• Steady uniform flow is a type of flow in which the conditions of flow do not alter with regard to the time at any given point on the passage.

 

Flow in duct

Steady Uniform flow

• Flow at a constant rate through a duct of uniform cross-section (The region close to the walls of the duct is disregarded)

Steady non-uniform flow

• Flow at a constant rate through a duct of non-uniform cross-section (tapering pipe)

Unsteady Uniform flow

• Flow at varying rates through a long straight pipe of uniform cross-section. (Again the region close to the walls is ignored.)

Unsteady non-uniform flow

• Flow at varying rates through a duct of a non-uniform cross-section.

Equivalent pipe system

• an equivalent pipe is defined as the pipe of uniform diameter having loss of head and discharge equal to the loss of head and discharge of a compound pipe consisting of several pipes of different lengths and diameters.

Or

•  if in the two pipe of the system having same flow rate and same friction loss then these systems of the pipe is said to be equivalent. The equivalent length method (L/D ratio) allows describing the pressure drop through the pipe due to friction loss corresponding to flow rate i.e. 

There are two combinations of equivalent pipe system:

• Pipes connected in series
• Pipes connected in parallel

For pipes connection in series:

• The discharge through all the pipes are the same and equivalent head loss is the sum of head loss in all the individual pipes.

• Qeq = Q1 = Q2 = … and heq = h1 + h2 + …..

• For pipes of same diameter of all the pipes equivalent length of the pipe is given by sum of length of all the pipes.

For pipes connected in parallel:

• The head loss through all the pipes are same and equivalent discharge is the sum of discharge in all the individual pipes.

• heq = h1 = h2 = …. and Qeq = Q1 + Q2 + …

Discharge through a channel, 

Turbulent flow 

• Turbulent flow occurs at relatively larger velocities and is characterized by chaotic behaviour, irregular motion, large mixing and eddies. For such flows, inertial effects are more pronounced than the viscous effects.

• Mathematically the velocity field of turbulent flow is represented as

V=V¯+V′

•  or the velocity fluctuates at small time scales around a large time-averaged velocity.

Similarly,  P=P¯+P′, T=T¯+T′etc.

Venturimeter

• As the fluid flows pressure drops along the direction of flow due to losses. Hence the more the losses along the flow the more will we be the pressure drop.
• Coefficient of discharge (Cd) is the measure of flow efficiency. It means higher the value of  Cd lesser will be the losses.
• Venturimeter is more efficient than the Orifice meter. Hence the coefficient of discharge is higher for Venturimeter than for Orifice meter.

Now,

• Parameter Reynolds number is used to characterize Laminar and Turbulent flow.
• If Re < 2100 for pipe flow the flow is laminar and if Re > 104 the flow is turbulent.

• ∵ (Cd)venturimeter  > (Cd)orifice meter 

• ∴ (ΔP)venturimeter < (ΔP)orific meter

Hence if Orific is replaced by a Venturimeter in a pipe then the pressure drop will decrease.

Venturimeter:

• A venturi meter is a device used for measuring the rate of flow of a fluid of a liquid flowing through a pipe
• The venturi meter always have a smaller convergent portion and larger divergent portion
• The size of the venturi meter is specified by its pipe diameter as well as throat diameter.

• This is done to ensures a rapid converging passage and a gradual diverging passage in the direction of flow to avoid the loss of energy due to the separation
• In the course of flow through the converging part, the velocity increases in the direction of flow according to the principle of continuity, while the pressure decreases according to Bernoulli’s theorem
• The velocity reaches its maximum value and pressure reaches its minimum value at the throat
• Subsequently, a decrease in the velocity and an increase in the pressure take place in course of flow through the divergent part
• The angle of convergence ≈ 20°, Angle of divergence = 6° - 7°. It should be not greater than 7° to avoid flow separation

Orifice meter:

• An orifice meter provides a simpler and cheaper arrangement for the measurement of flow through a pipe.
• An orifice meter is essentially a thin circular plate with a sharp-edged concentric circular hole in it.

• Cd is defined as the ratio of the actual flow and the ideal flow and is always less than one. 
• For orifice meter, the coefficient of discharge Cd depends on the shape of the nozzle, the ratio of pipe to nozzle diameter and the Reynolds number of the flow.

Steady and Unsteady flow:

• If the flow parameters such as velocity, acceleration, discharge do not change with time then flow is called steady flow, otherwise, flow is un-steady.

Gradually varied and Rapidly varied flow:

• If flow parameters such as velocity, acceleration, discharge changes with space, then flow is called non-uniform flow or varied flow. If the change in depth of flow is gradually along the length, then is it called gradually varied flow and if the change in depth of flow is sudden then it is called rapidly varied flow.

The examples for above kind of flows are given below:

Type of flow	Example
Steady and uniform flow	Flow in prismatic channel
Steady but gradually varied flow	Back water curve due to obstruction
Steady but rapidly varied flow	Hydraulic jump
Unsteady and Gradually varied flow	Flood flow in river
Unsteady and rapidly varied flow	Surge

• The surge means a sudden powerful forward or upward movement of water or powerful disturbance in water which could not be possible in gradually varied flow and steady flow.
• ∴ Development of surges in open channel is rapidly varied flow.

Channel slope	Profile notation	Flow depth	Froude number
Mild (M) (yn > yc)	M1 M2 M3	y > yn yc < y < yn y < yc	Fr < 1 Fr < 1 Fr > 1
Steep (S) (yc > yn)	S1 S2 S3	y > yc yn < y < yc y < yn	Fr < 1 Fr > 1 Fr > 1
Critical (C) (yn = yc)	C1 C3	y > yc y < yc	Fr < 1 Fr > 1
Horizontal (H) (So = 0)	H2 H3	y > yc y < yc	Fr < 1 Fr > 1
Adverse (A) (So < 0)	A2 A3	y > yc y < yc	Fr < 1 Fr > 1

Boundary layer: 

• A region in the immediate vicinity of the boundary surface in which the velocity of the flowing fluid increases gradually from zero at the boundary surface to the velocity of the main-stream is called the boundary layer.

• Flow separation occurs when the boundary layer travels far enough against an adverse pressure gradient that the speed of the boundary layer relative to the object falls almost to zero.

• It has been observed that the flow is reversed in the vicinity of the wall under certain conditions.

Seperation of boundary layer

• A favorable pressure gradient is one in which the pressure decreases in the flow direction (i.e., dp/dx < 0)
• It tends to overcome the slowing of fluid particles caused by friction in the boundary layer
• This pressure gradient arises when the freestream velocity U is increasing with x, for example, in the converging flow field in a nozzle
• On the other hand, an adverse pressure gradient is one in which pressure increases in the flow direction (i.e., dp/dx > 0)
• It will cause fluid particles in the boundary-layer to slow down at a greater rate than that due to boundary-layer friction alone
• If the adverse pressure gradient is severe enough, the fluid particles in the boundary layer will actually be brought to rest
• When this occurs, the particles will be forced away from the body surface (a phenomenon called flow separation) as they make room for following particles, ultimately leading to a wake in which flow is turbulent.

Specific energy: 

• It is defined as the energy of the flow in an open channel with respect to the bottom of the channel.

Hydraulic jump: 

• Whenever supercritical flow merges into a subcritical flow, a sudden jump is formed to reduce the energy of the water depth, is known as a hydraulic jump.

For a rectangular channel, energy loss is given as:

ΔE=(Y2−Y1)34Y1Y2

Here,  

Y2 = post jump depth, and Y1 = pre-jump depth

Froude number, Fr

`V/(gd)^(1/2)`

Fr=VgD√=Intertia_forceGravity_force−−−−−−−−−√

Where,

D = hydraulic depth = `A / T`

T = top width of the channel

Fr < 1 Subcritical or tranquil flow

Fr = 1 Critical flow

Fr > 1 Supercritical or rapid flow 

A hydraulic jump occurs when a supercritical stream meets the subcritical stream of sufficient depth.

Classification of hydraulic jumps are given below:

 If incoming Froude No. F is

Upstream Fr Description

< 1 Impossible jump

1 - 1.7 Undular jump

1.7 - 2.5 Weak jump

2.5 - 4.5 Oscillating jump

4.5 - 9 Steady jump

> 9 Strong jump

Friction and Pressure Drag

• A body moving through fluid experiences a drag force, which is usually divided into two components: frictional drag, and pressure drag.

• Frictional drag comes from friction between the fluid and the surfaces over which it is flowing. This friction is associated with the development of boundary layers, and it scales with Reynolds number.

• Pressure drag comes from the eddying motions that are set up in the fluid by the passage of the body. This drag is associated with the formation of a wake, which can be readily seen behind a passing boat, and it is usually less sensitive to Reynolds number than the frictional drag. It depends on the shape of the body.

• Frictional drag is important for attached flows (that is, there is no separation), and it is related to the surface area exposed to the flow. Pressure drag is important for separated flows, and it is related to the cross-sectional area of the body.

• For streamlined bodies (like a fish, or an airfoil at small angles of attack), frictional drag is the dominant source of air resistance. For a bluff body (like a brick, a cylinder, or an airfoil at large angles of attack), the dominant source of drag is pressure drag.

For flow over a sphere, Coefficient of drag is given by:

CD=24/Re

Where,

Re = Reynold’s number

Re=VD/ν

CD = Coefficient of drag

VT = Terminal velocity

ν = Kinematic viscosity of the fluid

Critical flow

• It is defined as the flow at which specific energy is minimum or the flow corresponding to critical depth is defined as critical flow.

• Froude number is 1 for critical flow.

Tranquil flow or streaming flow or sub-critical flow

• When the depth of a flow in a channel is greater than critical depth (hc), the flow is said to be sub-critical flow.
• Froude number is less than 1 for sub-critical flow.
• Torrential flow or shooting flow or super-critical flow

• When the depth of a flow in a channel is less than critical depth (hc), the flow is said to be sub-critical flow.
• Froude number is greater than 1 for sub-critical flow.

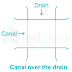
Vortex flow:

• The motion of a fluid in a curved path is known as vortex flow.
• 
  
• When a cylindrical vessel containing some liquid is rotated about its vertical axis, the vortex flow will be followed by liquid.
• 
  

Vortex motion is of two types:

1. Forced vortex:

• In the forced vortex, fluid moves on the curve under the influence of external torque.
• Due to the external torque, a forced vortex is a rotational flow.
• As there is the continuous expenditure of energy, Bernoulli's equation is not valid for forced vortex.
• For forced vortex, v = rω is applicable.
• Examples: 
• The flow of water through a runner of the turbine.
• Rotation of water in the washing machine.

2. Free vortex:

• When no external torque is required to rotate the fluid mass, that type of flow is called a free vortex.
• As there is no torque in the free vortex, so free vortex is an irrotational flow.
• For free vortex, a moment of momentum is constant i.e. vr = constant.
• Examples:
• The flow of liquid through a hole provided at the bottom of a container.
• Draining the bathtub.
• ∴vortex flow is both rotational and irrotational flow depending on the torque applied.

Velocity Potential function:

• 
  
• It is a scalar smooth function of space and time.
• It is defined for 1D, 2D and 3D flow.
• It is defined only for incompressible and irrotational flow
• It has to satisfy Laplace equation to be possible flow field.

u=−∂ϕ∂x

v=−∂ϕ∂y

w=−∂ϕ∂z

In order to predict the behaviour of a turbine working under varying condition of head, speed output etc, the results are expressed in terms of quantities which may be obtained when the head on the turbine is reduced to unity.

The following are three important unit quantities.

.

Unit speed	Speed of turbine working under  a unit head	Nu=NH√
Unit discharge	Discharge passing through a turbine  which is working under a unit head	Qu=QH√
Unit power	Power developed by a turbine working  under unit head	Pu=PH3/2 $\frac{{\mathrm{<br>}}^{}}{}$

Reciprocating pump:

• A reciprocating pump is a positive displacement pump where a certain volume of liquid is collected in an enclosed volume and is discharged using pressure to the required application.

Slip:

• The slip of a pump is defined as the difference between the theoretical discharge and the actual discharge of the pump. The actual discharge of a pump is less than the theoretical discharge due to leakage.
• Mathematically,
• slip = Qth- Qact
• where, Qth = Theoretical discharge, Qact = Actual discharge

Negative Slip:

• A negative slip of a pump is defined as the difference between the actual discharge and theoretical discharge of the pump.

Conditions negative slip is given as

• The delivery pipe is short.
• The suction pipe is long.
• The pump is running at high speed.
• Mathematically,
• Negative slip = Qact- Qth

For a single acting reciprocating pump 

The discharge through the pump is given by

Q=ALN/60

where, A = area of piston, L = stroke length, N = rpm of pump

:

NOTCH	WEIR
A notch may be defined as an opening provided on one side of a tank or reservoir with an upstream liquid level below the top edge of the opening.	A weir may be defined as a structure constructed across a river or a canal to store water on the upstream side.
The bottom edge of a notch over which water flows is known as sill or crest.	The top of the weir over which water flows is known as the crest.
A notch is usually made of a metallic plate.	A weir is made of a cemented concrete or masonry.
A notch is used to measure the small discharge of a small stream or canal.	A weir is used to measure the large discharge of rivers and large canals.
Notches are of small size.	Weirs are of big size.

assumption for Bernaullis equation